Chemistry Won’t Get Us to the Stars. What Will?
Astrophysicist Philip Lubin has said, “Chemistry will get you to Mars, but it won’t get you to the stars.” What did he mean by that? And, if chemistry won't get us to the stars, what will?
Mark Baumann
February 8, 2022
Philip Lubin, an astrophysicist at UC Santa Barbara, was quoted in a news update in Nature in 2017 as saying, “Chemistry will get you to Mars, but it won’t get you to the stars.” [1]Nature vol 542, pp 20-22 (2017) What did he mean by that? And, if chemistry won’t get us to the stars, what will? In this and future articles I will explore these questions.
This is part one of a four-part series on interstellar spacecraft.
Current Spacecraft Technology
Alpha Centauri is the star system that is nearest to ours. It is a triple star system with two Sun-like stars — Alpha Centauri A and B — and one red dwarf star — Alpha Centauri C, which is also called Proxima Centauri because it is currently (and will remain so for another 25,000 years) the closest one to us of the three stars. [2]The orbital period of Proxima Centauri, at about 547,000 years, is extremely long because it orbits around Alpha Centauri A and B at an extreme distance (thousands of AU). Eventually, it will orbit … Continue reading
And not only is the red dwarf star Proxima Centauri special for being closest to us, it is also home to a planet (called Proxima b, with a lowercase b) that is in the habitable zone of its star. This means that the natural first place to look for life beyond our solar system is Proxima Centauri.
How soon can we visit Proxima Centauri and look for alien life?
Proxima Centauri is 4.24 light years from us. It is our next-door neighbor. But, how far is 4.24 light years? In particular, how long would it take a spacecraft to get there?
Any spacecraft in low Earth orbit (such as the now-retired NASA Space Shuttle) must achieve a speed of about 7.8 km/s (roughly 17,500 mph) in order to remain in orbit. 17,500 mph is a lot faster than our highway speed limits of about 80 mph, but it is only 0.0026% the speed of light. At that speed, it would take the Space Shuttle 163,000 years to reach our next door neighbor. [3]Proxima Centauri is 4.25 light years away. At 0.0026% the speed of light, the journey would take 4.25/0.000026 = 163,460 years.
The Voyager 1 spacecraft was launched from Earth in 1977 and has since left our solar system. It is now the most distant human-made object. It is one of five spacecraft that have left our solar system (the others are Voyager 2, Pioneer 10 and 11, and New Horizons).
You can track the progress of Voyager 1 and 2 using this nifty dashboard from the Jet Propulsion Laboratory, which provides real-time information about their speed, location, and instrument status.
According to the dashboard, the current speed of Voyager 1 is about 17 km/s (38,000 mph); roughly twice that of a spacecraft in low Earth orbit like the Space Shuttle. Voyager has been sailing through space nonstop for over 40 years but, at that speed, it would take Voyager about another 75,000 years to cross the gap to Proxima Centauri. [4]The speed of light is about 300,000 km/s, so 17 km/s is 17/300,000 = 0.0057% the speed of light. At 0.0057% the speed of light, a journey of 4.25 light years would take 4.25/0.000057 = 74,560 years.

Parker Solar Probe trajectory | Image: Johns Hopkins Applied Physics Lab
The current speed record for a human-made craft is held by the Parker Solar Probe. It has traveled towards the Sun on shrinking orbits (see image), with the Sun’s gravity accelerating it each time. In December of 2021, the Parker Solar Probe passed through the Sun’s atmosphere, being the first spacecraft to touch the Sun.
In its gravity-boosted fly-bys of the Sun, the probe’s maximum speed has reached a blazing 163 km/s (364,660 mph). At that speed, it would take about 10 days for a spacecraft to travel the 91 million miles from the Earth to the Sun.
Even at that speed, though, it would take the probe over 7800 years to reach Proxima Centauri. [5]The speed of light is about 300,000 km/s, so 163 km/s is 163/300,000 = 0.054% the speed of light. At 0.054% the speed of light, a journey of 4.25 light years would take 4.25/0.00054 = 7870 years.
The earliest forms of writing appeared about 5500 years ago and therefore the journey to our next-door neighbor would take longer than all of recorded human history, even for humankind’s fastest-ever spacecraft.
Furthermore, due to the Sun’s gravity, the probe only accelerates when it gets closer to the Sun (like a ball rolling downhill). That’s how it achieved such high speeds in the first place. As it gets farther from the Sun, it decelerates (like a ball rolling uphill). So even though it has reached a speed of 364,550 mph, if it were to climb out of the Sun’s gravity and leave the solar system, its speed for the trip to Proxima Centauri would be significantly less.
That’s the current state of the art in high speed human-made vehicles. But, could the rockets of the future potentially cover the distance to our next-door neighbor on time scales shorter than all of recorded human history?
Ideally, could we design a spacecraft that can travel to Proxima Centauri in less than a human lifetime?
Can Rocket Technology Get Us to the Stars, Eventually?
In discussing whether conventional rocket technology could theoretically ever get us to the stars, a simple application of the famous Tsiolkovsky rocket equation (informally the delta-v equation) is very informative.
The delta-v rocket equation was derived independently by British mathematician William Moore in 1810, Russian rocket scientist Konstantin Tsiolkovsky (for whom the equation is named) in 1903, American engineer Robert Goddard (for whom NASA’s Goddard Space Flight Center is named) in 1912, and German physicist Hermann Oberth in 1920.
The equation is derived from a simple application of the conservation of momentum: as the rocket exhaust leaves the back of the rocket, it carries with it some momentum (opposite the direction that the rocket is going), and that in turn gives the rocket an equal-but-opposite momentum boost forward (in the same direction that the rocket is going). In that way the total momentum is left unchanged, in accordance with the well-established law of conservation of momentum.

Propelling yourself by throwing bricks out of a wagon uses the same principle as rocket propulsion
This is analogous to sitting in a wagon full of bricks and propelling yourself forward by throwing bricks out of the back of the wagon. (I’m using this example because I still remember being given that problem to solve in my freshman physics course in college. That I still remember it 20 years later is a testament to how memorable was my struggle to solve it. Now that I realize my professor was asking us to derive the rocket equation, I don’t feel so bad about struggling with it when I was just starting out in college physics.)
This argument is also equivalent to Newton’s Third Law: for every action, there’s an equal and opposite reaction. Throwing a brick out of the back of the wagon causes the wagon to move forward. Likewise with a rocket: pushing rocket exhaust out of the back of the rocket pushes the rocket forward. As the character of Cooper (played by Matthew McConaughey) says in the movie Interstellar: “Newton’s Third Law [says] you can’t get anywhere without leaving something behind.”
The delta-v equation can be thought of as a machine (a “black box”) that takes three inputs:
- The exhaust velocity,
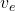 — which is created by the chemical reaction of burning the rocket fuel and then focusing the chemical products through an exhaust nozzle (for instance, a de Laval nozzle),
— which is created by the chemical reaction of burning the rocket fuel and then focusing the chemical products through an exhaust nozzle (for instance, a de Laval nozzle), - the starting mass of the rocket plus its fuel,
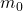 ,
, - and the final mass of the rocket after all of its fuel has been expended,
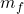 .
.
Given those three inputs, the equation then returns the change in velocity ![]() (the delta-v as the equation is informally called) of the rocket.
(the delta-v as the equation is informally called) of the rocket.
In case you want to see what’s inside the black box, here’s what the equation looks like:
![]()
Kulkarni et. al.[6]Kulkarni, Lubin, and Zhang, Astronomical Journal vol 155 no 4, 2018 perform an interesting calculation using this equation. Let’s make the rocket as small as possible and use as much fuel as possible to find the highest theoretical speed to which we can accelerate the rocket. In that vein, suppose the “rocket” is a single proton. And suppose that the propellant is the mass of the entire visible universe!
In other words, if we converted the entire mass of the visible universe into rocket fuel, how fast could we get a single proton moving using a conventional rocket? That should give us some sense of the theoretical upper limit of a rocket’s speed.
To that end, Kulkarni et. al. use a typical rocket exhaust velocity of ![]() km/s (
km/s (![]() in the rocket equation). I’m going to use
in the rocket equation). I’m going to use ![]() km/s since that is a theoretical upper limit for exhaust velocity of a chemical rocket. The mass of a proton is about
km/s since that is a theoretical upper limit for exhaust velocity of a chemical rocket. The mass of a proton is about ![]() grams (
grams (![]() in the rocket equation). The mass of the visible universe is about
in the rocket equation). The mass of the visible universe is about ![]() grams (
grams (![]() ).
).
Plug these numbers into the delta-v formula and we find the proton’s final velocity as 921 km/s. That’s fast! For comparison, recall that the Voyager 1 spacecraft that left our solar system is traveling about 17 km/s, and the Parker Solar Probe reached a max speed of 163 km/s. This is more than five times as fast as the Parker Solar Probe and more than 50 times as fast as Voyager 1. However, 921 km/s is still just 0.3% the speed of light.[7]Once speeds become relativistic (at least about 1% of the speed of light), a relativistic version of the rocket equation is required. Since we are still just at 0.3% the speed of light, the … Continue reading
At 0.3% the speed of light, it would take a spacecraft about 1417 years to get to Proxima Centauri.[8]Proxima Centauri is 4.25 light years away. At 0.3% the speed of light the journey would take 4.25/0.003 = 1417 years. And that’s the theoretical best case for chemical rockets.
So even the mass of the entire visible universe isn’t sufficient fuel to accelerate even a single proton to “relativistic” (near-light) speeds using conventional rockets. And since conventional rocket technology is powered by chemical reactions, this is why Lubin said, “Chemistry will get you to Mars, but it won’t get you to the stars” … Unless we’re willing to wait more than a millennium.
What About Fusion-Powered Spacecraft?
If chemical reactions are insufficient, what about nuclear reactions?
A fusion reaction produces a tremendous amount of energy in the form of a plasma (ultra hot gas). The resulting plasma could be constrained by a magnetic field so that it can escape in only one direction, like a rocket exhaust. Note that this form of propulsion also follows the Tsiokolvsky rocket equation. However, the exhaust velocity (![]() ) can be much higher than for a chemical rocket, and therefore it won’t have the same limitations in achieving relativistic speeds.
) can be much higher than for a chemical rocket, and therefore it won’t have the same limitations in achieving relativistic speeds.
A Very Brief Synopsis of Nuclear Fusion
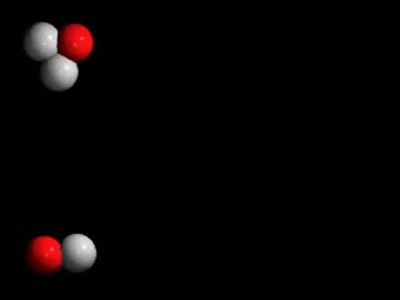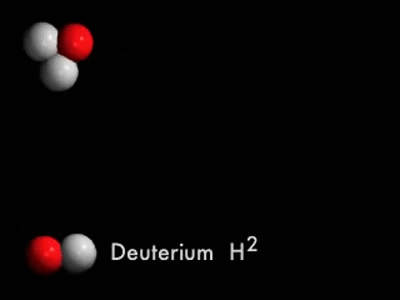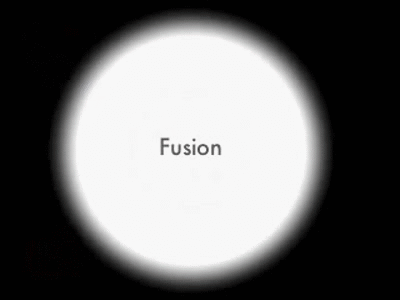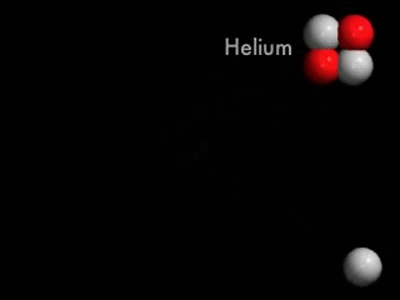
Nuclear fusion is the process by which two nuclei slam together and stick together, creating a heavier (larger) nucleus. For example, consider two hydrogen nuclei, each with one proton. If they slam into each other and stick together to form a new nucleus, the new nucleus will have two protons. Since the number of protons determines an atom’s position on the periodic table, the new nucleus is a helium nucleus.
Atomic nuclei are all positively charged. And like-charges repel (via the electric force). Therefore, two atomic nuclei are going to repel because they are both positive. And the closer they get, the more stubbornly they repel each other. Therefore, it is very hard to force two nuclei to meet. They must be going extremely fast, which means that they must belong to a very hot gas. How hot? About a hundred million degrees will do the trick.
Temperatures inside stars are high enough that nuclei are moving fast enough that they can slam together. Once they do so by overcoming the electrical repulsion — the so-called Coulomb barrier — the strong nuclear force is then able to take over. The strong nuclear force is another fundamental force of nature; it holds a nucleus together and it operates only over extremely short ranges (i.e., ranges of about the width of a nucleus; or, a few femtometers; 1 fm = 10-15 m).
Although it requires a very large input of energy to slam two nuclei together to make a bigger nucleus (since the nuclei must be moving so fast to overcome the Coulomb barrier), the process also releases a tremendous amount of energy. Fusion is an exothermic reaction (up to a certain point on the periodic table), meaning you get more energy out of it than you put into it.
Fusion is what powers stars, like our Sun, and makes them shine. Without nuclear fusion, there would be no light energy arriving on Earth from the Sun, and therefore no photosynthesis; Earth would be a radically different place.
Fusion-Powered Spacecraft
Starting at the beginning of the periodic table, the simplest form of nuclear fusion entails slamming two hydrogens together; or, in the next simplest case, a hydrogen and a helium. Because there are various isotopes of hydrogen and helium, this works out to more than just two cases. For powering a spacecraft, there are three possible nuclear fusion reactions worth considering:
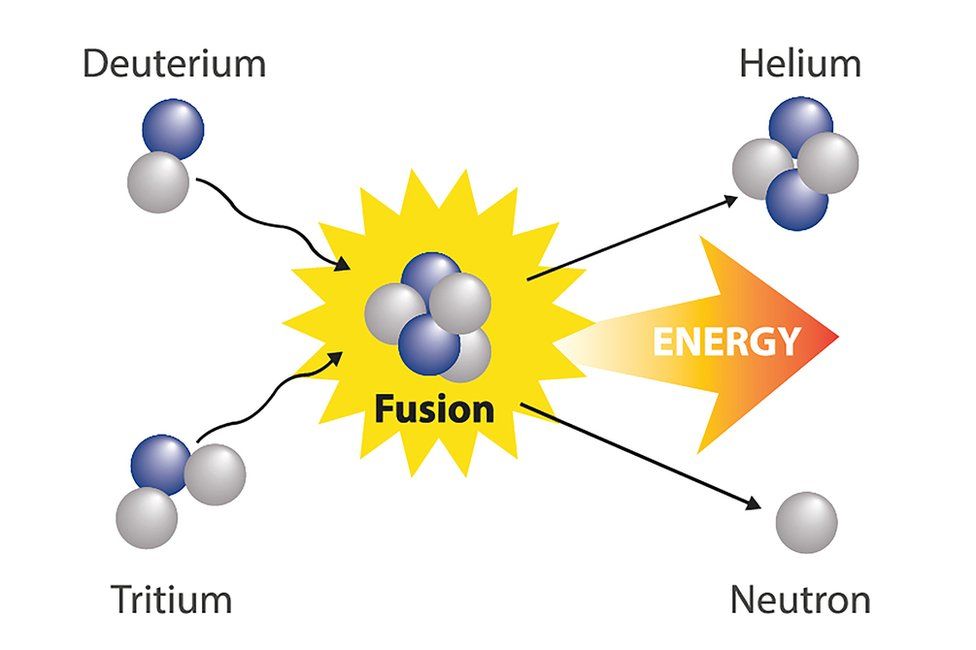
DT Fusion | Image: bbc.com
- Deuterium-Tritium (“DT“)Hydrogen, the simplest element, contains just a single proton in its nucleus.Deuterium, also called heavy hydrogen, is a hydrogen nucleus that contains both a proton and a neutron.
Tritium is an even heavier version of hydrogen, with a proton and two neutrons in its nucleus. Tritium is unstable and prone to radioactive decay; it has a half-life of about 12 years, which means that in 12 years about one-half of the Tritium will decay naturally.
The 12-year half life of tritium is problematic for a long voyage, since the trip time would be severely limited by the very short shelf life of the fuel. Alternatively, the spacecraft mission could be one in which it accelerates at the start and then rides out the rest of the voyage without the ability to decelerate; in other words, a fly-by mission.
Another drawback to DT fusion is that each time a deuterium fuses with a tritium, the reaction releases a neutron that zips along at close to the speed of light (you can see this neutron product in the fusion animation above). The neutron alone carries away 80% of the energy produced by the fusion reaction, which is mostly wasted energy since neutrons are harder to corral than charged particles — since neutrons have no charge, they are unaffected by electric and magnetic fields. Furthermore, all of those high-energy neutrons are potentially catastrophically dangerous to the rest of the spacecraft unless they are properly absorbed by a shield or deflected off into space.
- Deuterium-Helium 3 (“DHe3“)Helium-3 is a helium nucleus with two protons and one neutron. It is a stable isotope, meaning it doesn’t decay radioactively like tritium.Advantages of DHe3 over DT are that the reactants are stable (unlimited shelf life) and there’s no energetic neutron byproduct. However, DHe3 has some disadvantages. The Coulomb barrier is bigger and so the energy input to start a DHe3 reaction is higher than for DT. Also, the DHe3 reaction can produce tritium nuclei, and those will in turn react with the deuterium fuel, resulting in DT reactions as a side product that ultimately produce those undesirable high-energy neutrons.
And perhaps the biggest disadvantage of DHe3 is that Helium-3 is virtually nonexistent on Earth. We could mine some on the Moon, but to acquire the extremely large quantity of Helium-3 needed for spacecraft fuel we would have to mine it from other planets in our solar system, such as the gas giants. At this time, it’s not possible to mine anything on the Moon let alone other gas giants. However, perhaps in a century this could be possible.
- Deuterium-Deuterium (“DD“)As the name implies, a DD reaction is one in which two deuterium (heavy hydrogen) nuclei are slammed together.DD, like DT, produces a high-energy neutron that carries a significant fraction of the output energy, and so it shares the same disadvantages of wasted energy and dangerous by-products.Perhaps the biggest advantage of DD is that it uses only deuterium for fuel. Deuterium is stable and it’s common enough that it can be relatively easily obtained on Earth.
Water consists of two hydrogen atoms and an oxygen atom combined via covalent bonds. Out of every 5000 hydrogen atoms among water molecules in the ocean, one of them is deuterium. A water molecule that uses deuterium in place of its hydrogen atoms (written D2O instead of H2O) is commonly called heavy water (or semi-heavy water if only one of its hydrogen atoms is a deuterium, which is written HDO). Therefore, deuterium can be acquired by distilling heavy water out of the oceans. There are multiple processes for doing this that are in use today since heavy water is routinely used in science experiments around the globe.
Project Daedalus
In the 1970’s, there was a concerted effort to design a fusion-powered spacecraft. It was called Project Daedalus, named for the engineer from Greek myth that built the Minotaur’s labyrinth and created wings so that he and his son could fly. The design process lasted about 5 years and was organized by the British Interplanetary Society. [9]Project Daedalus : the final report on the BIS starship study, Bond, Alan and Project Daedalus Study Group, Journal of the British Interplanetary Society Supplement, 1978

Comparison of Daedalus and Saturn V rocket; the spherical components are the fuel tanks | Image: Adrian Mann
Daedalus would be a massive spacecraft with an overall length of 190 meters and a weight of 54,000 metric tons when fully loaded with fuel. For comparison, NASA’s Saturn V rocket (the largest rocket ever launched into space) was about 3000 metric tons including fuel (see image for size comparison).
The Daedalus team proposed using DHe3 fusion for propulsion. The hot plasma created by the fusion reaction could produce an exhaust velocity ![]() of about 10,000 km/s. Compare that with the upper limit
of about 10,000 km/s. Compare that with the upper limit ![]() for the chemical rocket that we used in the rocket equation earlier, which was just 5 km/s!
for the chemical rocket that we used in the rocket equation earlier, which was just 5 km/s!
Daedalus would have two-stage propulsion; stage one would fire for two years and accelerate the spacecraft to 7% the speed of light. Stage one would then be jettisoned, reducing the mass significantly, and the second stage would fire for just under two more years until Daedalus reached 12% the speed of light. At this point, Daedalus will have expended all of its fuel. Therefore, it wouldn’t have the ability to decelerate and the Daedalus mission would be a fly-by mission by design.[10]Interstellar Travel: A Review for Astronomers, Crawford I.A., Quarterly Journal of the Royal Astronomical Society, Vol. 31, p.379-381
To achieve a speed of 12% the speed of light, however, would require 50,000 metric tons of propellant — a significant fraction of the spacecraft’s overall mass of 54,000 metric tons. Notice the huge spherical fuel tanks in the image of Daedalus above and you get a sense of how much of the spacecraft’s total mass is just propellant. For DHe3 fusion, half of that propellant is deuterium and half is Helium-3. And as discussed above, Helium-3 is very hard to come by. Only a place like Jupiter could serve as a sufficient source for acquiring that much Helium-3.
So while a fusion-powered craft like Daedalus could, in principle, accelerate to relativistic speeds, to build such a massive craft and acquire the fuel for it is probably at least 100 years beyond our reach right now.
Project Icarus
Project Daedalus was revisited 30 years later, in 2009. This time it was named Project Icarus, for the son of the mythical Daedalus. Once again organized by the British Interplanetary Society, planning on Project Icarus lasted 10 years.
Numerous design ideas were put forth but the participants were unable to agree on a single design. Therefore they held an internal competition. The winning design of the competition was later shown to scale poorly and therefore was ultimately eliminated as a viable solution. Only one design from the project made it to publication. It is called Icarus Firefly and was published in 2020 [11]Project Icarus: Designing a Fusion Powered Interstellar Probe, Swinney, R.W. and Freeland II, R.M. and Lamontagne, M., Acta Futura: 47-59 (2020)
Unlike Project Daedalus, which proposed using DHe3 fusion that requires currently unobtainable Helium-3 fuel, Icarus Firefly proposed using DD fusion since deuterium is widely available from distilling seawater, as mentioned.
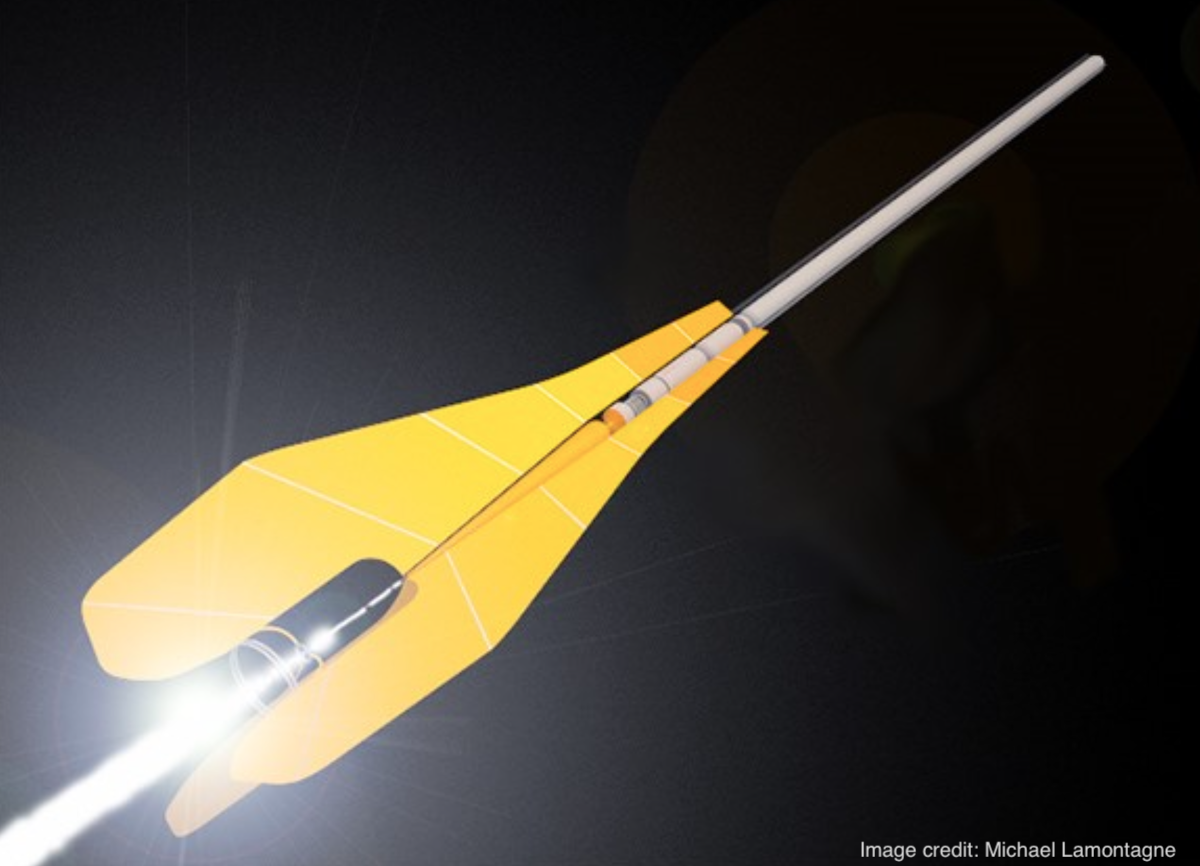
A major byproduct of DD fusion are highly-energetic neutrons. The shielding required to protect the fuel and scientific payload would add a significant amount of mass to the spacecraft. Therefore, the shape of the spacecraft is designed to be long and thin so that it would radiate the neutrons outward into space in a mostly cylindrical pattern. Only a small shield is therefore required to protect the fuel and scientific payload. This design choice resulted in a spacecraft that is shaped like a lawn dart and is an incredible 750 m long; more than twice the length of a modern aircraft carrier.

Icarus Firefly | Image: Michael Lamontagne
The input power to start the fusion reaction in Firefly is a whopping 1170 GW (gigawatts). For comparison, at any given moment, the entire United States uses on average about 482 GW.[12]In 2018, the total US consumption of electricity for the year was 4222.5 TWh (terawatt hours) according to a report from the U.S. Department of Energy. Divide this by the number of hours in a year … Continue reading
In its final design, Icarus Firefly would accelerate over a period of 10 years, reaching a maximum speed of 5% the speed of light. It would cruise for 85 years, then decelerate over a period of 5 years to arrive in orbit around Alpha Centauri.
In total, Icarus Firefly would make a 100 year voyage to Alpha Centauri.
Summary
To date, human-made craft have achieved a highest speed of 0.054% the speed of light. The Parker Solar Probe achieved that velocity through a combination of chemical rocketry and gravitational assistance from the Sun. At that speed, the journey to Alpha Centauri would take 7800 years, longer than all of recorded history.
The theoretical maximum velocity for a chemical propelled rocket, calculated using the Tsiolkovsky rocket equation, is 0.3% the speed of light. At that speed, the journey to Alpha Centauri would take 1417 years.
To get to Alpha Centauri in under a millennium, we will need to look at other methods of propulsion besides chemical-reaction-based propulsion.
Project Daedalus, a proposed DHe3 fusion powered spacecraft, could in principle reach 12% the speed of light. The vast majority of the spacecraft’s mass would be fuel. All of its fuel would be used up to accelerate, making deceleration impossible and so it would be a fly-by mission. Since Daedalus uses DHe3 fusion, it would require a significant amount of Helium-3 fuel that cannot be obtained on Earth. Until we develop the ability to mine other planets, a DHe3-propelled spacecraft isn’t a viable option.
Icarus Firefly, a more recently proposed fusion spacecraft, uses DD reactions instead of DHe3, and so the fuel is readily available. The flight time for Firefly from Earth to Alpha Centauri is 100 years, longer than an average human lifespan.
Conclusion
Between chemical rockets and nuclear fusion, using fuel that can be readily obtained and technology that exists today, we do not have a viable design for reaching Alpha Centauri in less than a human lifespan.
Are we out of luck? Not quite. There are more options that I will explore in the next three articles. In the next article, I will be discussing another possibility: nuclear pulse propulsion, which involves repurposing our nuclear weapons arsenal for spacecraft propulsion.
Footnotes
| ↑1 | Nature vol 542, pp 20-22 (2017) |
|---|---|
| ↑2 | The orbital period of Proxima Centauri, at about 547,000 years, is extremely long because it orbits around Alpha Centauri A and B at an extreme distance (thousands of AU). Eventually, it will orbit around to the other side of A and B, making A and B the closest stars to us. A and B are closer together and so their orbital period is smaller, at about 80 years. Therefore, for half their orbit — every 40 years — they will trade off being the closest to us. But none of that will happen for another 25,000 years. |
| ↑3 | Proxima Centauri is 4.25 light years away. At 0.0026% the speed of light, the journey would take 4.25/0.000026 = 163,460 years. |
| ↑4 | The speed of light is about 300,000 km/s, so 17 km/s is 17/300,000 = 0.0057% the speed of light. At 0.0057% the speed of light, a journey of 4.25 light years would take 4.25/0.000057 = 74,560 years. |
| ↑5 | The speed of light is about 300,000 km/s, so 163 km/s is 163/300,000 = 0.054% the speed of light. At 0.054% the speed of light, a journey of 4.25 light years would take 4.25/0.00054 = 7870 years. |
| ↑6 | Kulkarni, Lubin, and Zhang, Astronomical Journal vol 155 no 4, 2018 |
| ↑7 | Once speeds become relativistic (at least about 1% of the speed of light), a relativistic version of the rocket equation is required. Since we are still just at 0.3% the speed of light, the non-relativistic Tsiokolvsky equation above is still an appropriate equation. |
| ↑8 | Proxima Centauri is 4.25 light years away. At 0.3% the speed of light the journey would take 4.25/0.003 = 1417 years. |
| ↑9 | Project Daedalus : the final report on the BIS starship study, Bond, Alan and Project Daedalus Study Group, Journal of the British Interplanetary Society Supplement, 1978 |
| ↑10 | Interstellar Travel: A Review for Astronomers, Crawford I.A., Quarterly Journal of the Royal Astronomical Society, Vol. 31, p.379-381 |
| ↑11 | Project Icarus: Designing a Fusion Powered Interstellar Probe, Swinney, R.W. and Freeland II, R.M. and Lamontagne, M., Acta Futura: 47-59 (2020) |
| ↑12 | In 2018, the total US consumption of electricity for the year was 4222.5 TWh (terawatt hours) according to a report from the U.S. Department of Energy. Divide this by the number of hours in a year (365.25 * 24 = 8766) and that’s an average of 482 GW (gigawatts) being used continuously by the US over the course of a year. Note that this average includes both day and night, so the actual consumption will be higher than 482 GW during the day and lower than 482 GW at night. |
